EC.4. Tools, Tasks, and Talk as Essential Pedagogies for Meaningful Mathematics
EC.4. Tools, Tasks, and Talk as Essential Pedagogies for Meaningful Mathematics
Well-prepared beginning teachers of mathematics at the early childhood level intentionally plan for and use tools, tasks, and talk as pedagogies for young children’s engagement in meaningful mathematics. [Elaboration of C.2.2 and C.2.3]
Effective teaching entails meeting children where they are mathematically on a learning trajectory and employing instructional pedagogies that utilize tools, tasks, and talk to support advancement in children’s mathematical understanding and skills. Candidates in effective teacher preparation programs learn to ask and answer these fundamental questions: Is this (child) group of children on the learning trajectory as expected for (his or her) their ages and grades? If not, where are they on the trajectory? Where do they need to move next mathematically? How can I, as their teacher, provide instructional experiences that help them progress in their understanding and use of mathematics? What instructional tasks, tools, and activities might be most beneficial to support their learning? How can I engage these young learners in mathematical conversations and discourse that helps them connect their experiences and informal language with the world of mathematics? What questions should I ask them to draw out their observations and engage them in mathematical talk?
Well-prepared beginners select tasks purposefully and prompt children to use tools in solving mathematical problems to support children’s progress on specific learning trajectories. They know that not all tasks provide the same opportunities for developing children’s thinking and learning and that even young children need regular experiences with high-level tasks (Hiebert & Wearne, 1993; Stein et al., 1996). They also know that young children are good problem solvers and learn through problem-solving experiences (Cai, 2003; Moser & Carpenter, 1982). These teachers see their roles as helping children mathematize their worlds while nurturing understanding of mathematical concepts and relationships and developing language to talk about those emerging observations. They situate mathematical tasks in children's ways of knowing and learning, including attending to children's cultures, languages, genders, socioeconomic statuses, cognitive and physical abilities, funds of knowledge, and personal interests. In addition, these teachers know not to rush children toward procedural fluency; they recognize that such fluency builds from conceptual understanding through use of informal reasoning strategies in solving problems and that it develops over long periods of time, from months to years (NCTM, 2014a; NRC, 2001a).
Effective teachers learn to see and view mathematics through the eyes of their students, especially in the early childhood years when children's conceptions can be quite different from those of the teacher (see Vignette 4.2). Teachers know that all mathematical ideas are abstract, and learners have access to those ideas only through representations (NRC, 2001a). This challenge is especially prevalent among young children who are newly experiencing ways to mathematize their experiences and observations with physical objects, verbal analogies, and drawings as well as with invented and standard symbolic representations. Thus, well-prepared beginners demonstrate their own representational competence in using physical, visual, verbal, symbolic, and contextual representations appropriate for early mathematics; they recognize that these representations are foundational ideas for later mathematics. In addition, they know how to strategically use mathematical tools (e.g., part-whole mats, number bonds, Rekenreks/math racks, ten frames, bead strings, and tape diagrams) to develop and advance children’s mathematical understanding and skills.
The work of teaching is complex, regardless of the age of the students. This work includes not only attention to tasks and tools for mathematical inquiries but also attention to involving young learners in meaningful mathematical talk or discourse when they engage in structured mathematical activities as well as in play (Van Oers, 2010). “Mathematical discourse includes the purposeful exchange of ideas through classroom discussion, as well as through other forms of verbal, visual, and written communication” (NCTM, 2014a, p. 29). Mathematics talk is particularly important for young children, given their limited but emerging abilities to write words and use mathematical symbols. Well-prepared beginners know that young children need many opportunities to talk about their mathematical observations and emerging ideas as well as to listen to and learn from their peers. Thus, well-prepared beginners become skillful in noticing and eliciting children’s thinking and then engaging them in learner-focused dialogue, or mathematics talk, that links children’s informal experiences and words to more formal mathematical ideas, using language appropriate for the particular learners (Rudd, Lambert, Satterwhite, & Zaier, 2008; Whitin & Whitin, 2000).
Vignette 4.2. Building From What Children Understand Mathematically
The candidates in a mathematics methods course were asked to read the short narrative shown below and analyze the thinking of two children, Ethan and Morgan.
----------------------------------------------------------------
Both Ethan and Morgan are 4 years old and are enrolled in a preschool program. This is Morgan’s second year in the program, whereas Ethan is new to the program this year. One day the teacher checked their subitizing abilities through an informal assessment. Working with one child at a time, she flashed each dot pattern shown below for a few seconds and then asked the child, “How many dots did you see?” If the child hesitated or was unsure, the pattern was shown again or given to the child to examine more closely. Then the child was asked to explain what he or she saw and how he or she determined the total number of dots.
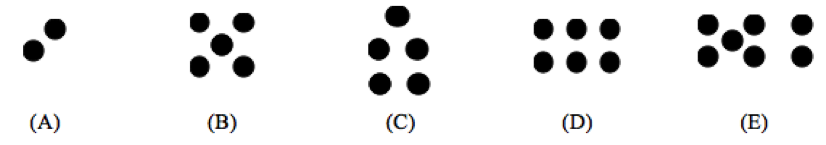
Ethan was able to identify two dots at a glance (Pattern A) but needed to count the other sets of dots by ones by touching each dot with his finger. For Pattern C, he guessed, “Eight,” and for Pattern E he said, “I call that one 11.” When he was handed the dot patterns, he counted the dots by ones and was able to recite the number names in the correct order, but he often had to recount the sets because he often lost track of which dots he had counted and which still needed to be counted. He demonstrated a connection between his counting and the cardinality of each set.
Morgan was able to quickly identify the total number of dots in each set without having to count by ones. For Patterns A, B, and D, the familiar dice configurations, she very quickly identified the total number of dots as two, five, and six. When asked, “How do you know it’s five?” for Pattern B, she replied, “Cuz there’s two and two and one in the middle. Five!” For Pattern D, she explained, “Cuz they’re in the right order.” She hesitated for only a moment with Pattern C and then said it was five dots and explained, “‘Cuz these are four and this is five, but not in the middle” as she pointed to the familiar arrangement of four dots on the bottom of the card and then pointed to the one dot on top.
----------------------------------------------------------------
Working in pairs, the candidates placed each child's performance on the subitizing learning trajectory and then made instructional suggestions for advancing the learning of each child. This activity included identifying specific tasks and useful representations, formulating purposeful questions to ask the children, and articulating the mathematical knowledge and reasoning being targeted with each task. Then the whole class reconvened and collectively reached consensus on each child’s learning-trajectory placement and next instructional steps. (Based on Huinker, 2011)
